Mathematics for elementary students has been changing dramatically for the last 20 years.  Gone are the days where students in first through fifth grades learn addition, subtraction, multiplication and division with fractions and decimals thrown in by fifth grade.  Students were asked to memorize their multiplication tables, quickly add and subtract double-digit numbers without using their fingers, and complete long division problems using prescribed steps.

For better or for worse, curriculum gurus developed a spiral type of learning that includes addition, subtraction, multiplication, division, fractions, decimals, algebra, geometry, complex and multi-step word problems and higher order thinking skills.  The idea is that students will be introduced to each type of mathematics from Kindergarten through Sixth grade.  Each grade year, students will learn and be able to apply deeper and more complex strategies to solve problems.  The goal is by Sixth grade for students to be exposed to and understand the complex nature of mathematics.  Some educators feel that this is great because the United States has fallen behind in terms of math test scores throughout the world, while other educators worry that students in this new curriculum never get a chance to master anything.

Whichever school of thought you may lean towards, I believe this new curriculum is here to stay, at least in the foreseeable future.  The School District of Philadelphia recently purchased new math curriculum from Pearson called enVisionmath.  It uses the same “spiral” learning as Chicago math introduced years ago.   Our students struggle with this curriculum because 1) it assumes fourth graders have been using envision math for their elementary years and 2) it includes very abstract concepts and skills for students who are not developmentally equipped to solve complex, multi-step problems when they have not mastered their addition, subtraction, and multiplication skills.  Let me be clear – students understand for the most part how to add and subtract up to double and triple digits.   They understand that multiplying means to add groups of numbers.  What they do not have is the hours of focus and practice it takes to 1) have number sense in which, for instance, students see immediately that 345 + 135 cannot equal 4, 080 and 2) memorize their multiplication facts so that dividing and multiplying fractions become skills they can succeed in.

I currently teach fourth graders in North Philadelphia.  My student population is made up of 27% African American, 63% Hispanic/Latino, 3% White, 1% Asian, and 6% Multi Racial/Other.  We are a Title I school with 100% recorded as economically disadvantaged.  My students have a difficult time focusing on their lessons, completing home and school work, and self-monitoring their behavior.  I find when teaching math that my students get upset when presented with new concepts that assume they have mastered basic math skills.  Our curriculum also assumes students have prior experience with the curriculum (it is our second year using this curriculum) and have been successful.  I find that many times, my students do not know what to ask when they do not understand a concept.  It seems that most do not have the basic terminology to articulate questions they have about the math concepts are learning.

Have you ever seen students making fortune-tellers in your classroom?  Students in my class this year make them all the time.  I introduced an “origami book,” which is a fun way to organize information about non-fiction texts.  Now I find my students making 3-D shapes in the classroom such as boxes and shapes that involve several pieces of paper locked together like a pinwheel.  Geometry can be fun and engaging for elementary students.  Identifying shapes, understanding angles and degrees of a circle, identifying triangles, etc. give students a break from the usual adding, subtracting, multiplying and dividing.  For our students in the Philadelphia School District, providing additional practice and hands on activities will give them a chance to play with these concepts and hopefully gain a deeper understanding.

Norma Boakes wrote that spatial visualization is needed if students are going to understand shapes and structures; they need to spend time exploring and developing their spatial skills.  One way to do this is through origami.  Origami involves a student “following a construction process moving a two-dimensional square into a variety of three-dimensional shapes and figures.” (Boakes 2)

This unit uses the idea of engaging students by uses a simple manipulative – paper.  Students will be able to create shapes, both two and three-dimensional to develop an understanding of symmetry, angles, fractions, and measurement.

Using paper, a manipulative, that students can work and practice with, will help them to better grasp concepts such as measuring the degrees of a circle.  Designing problems and projects around geometric concepts will help students to better understand these abstract concepts.

Brief History of Paper

There are a few books out that look at history through objects or food.  It is a fascinating way to chart history through time without necessarily charting wars and battles.  Mark Kurlansky has written a few of these.  Salt: A World History, Cod: A Biography of the Fish that Changed the World, The Big Oyster: History on the Half Shell, and Milk! and A 10,000-Year Food Fracas are just a few of his books that look at the history of these items and how the need, distribution, sale, use, and quest for them have changed the world.

Paper: Paging Through History was also written by Kurlansky in 2016.  Paper as we know and use it today is a relatively new technology.  Paper satisfied a need in society – a need to record information.  As governments grew and changed and as businesses and trade grew and changed, the need to record and keep records of transactions and laws became important.

Kurlansky wrote that the Chinese invented papermaking.  That is not to say that a type or form of paper was not being used in other parts of the world around the same time.  Evidence shows that people were writing on materials such as clay, stone, papyrus and parchment.

At one point, the mark of an advanced civilization was one that made paper.  As paper became the cheaper option (much cheaper that papyrus, a water plant, which could only be grown along the Nile and parchment, which is made from the skins of sheep, goats, etc.), its use spread throughout the world.  As merchants from the East traveled throughout Asia, paper was traded and eventually paper mills grew around the world changing the economy of those towns that settled near a good source of running water.  Because papyrus was unique to Egypt, it became a valuable commercial product that was shipped throughout the world.

The papyrus reed was peeled and once the outer layer was removed, there were about twenty inner layers that would be unrolled and laid out flat.  The layers were “woven” together, the second set laid at a 90-degree angle from the first set underneath.  Water was used to moisten the sheets and then they were pressed together with weights for a few hours.  The reeds, when cut, had a sticky sap that served as the glue that kept the layers together and if needed, a flour paste was used.  The sheets were then rubbed with a stone, piece of ivory, or shell until they were smooth and the layers did not create grooves that a stylus could move across the sheet.

Parchment was made from the skins of sheep, goats, and cows.  Vellum, a finer quality of parchment, was made from the skins of calves.  The process was tedious: after being flayed, the skin is soaked in water for a day.  The skin was soaked in a dehairing liquid, which eventually included lime, for eight days.  It had to be stirred a couple of times a day and you had to be careful not to soak the skin too long because it weakened the skin.  Next it was stretched out on a stretching frame by wrapping small, smooth rocks in the skins with rope or leather strips. The skin would be scraped to remove the last of the hair and get the skin the right thickness.

Paper is made by “breaking wood or fabric down into its cellulose fibers, diluting them with water, and passing the resulting liquid over a screen so that it randomly weaves and forms a sheet.” (Kurlansky xv)  Different trees and plants were used to make paper so through the last two to three centuries, paper has evolved from a thicker, coarser paper to the thin, smooth paper that we use today.  Paper was slow to become popular in Europe, it was felt that important and religious books should be written on parchment because they would last longer than books made of paper.

Making paper was not necessarily easier or faster than making papyrus or parchment.  In fourteenth century Europe, papermaking was common and wherever there was a river with clean water, a downhill run or swift moving current, and a town of people who could provide rags, there was a paper mill.  As the need for paper grew, workers, who did not have fixed hours, might work all night.  Apprentice paper workers, or children, “who were small enough to crawl into vats, scrubbed the hammers and the equipment clean” during the night when the mill was closed.  (Kurlansky 96)

With our new digital technologies, one might think that paper is seeing its last days.  Kurlansky would agree that paper might not be here forever.  But he does feel it is

more secure than electronic messages.  “Electronic messages can be hacked, accessed and reconstructed.” (Kurlansky 334)  If origami continues to be popular and used in education, health care and science, then paper will continue being manufactured in the future.

Timeline

To give a perspective of how paper has evolved to today, here is a timeline of major events known throughout history taken from Paper: Paging Through History:

3000 BCE      Oldest papyrus found – a blank scroll in a tomb at Saqquara, near Cairo

500 BCE         Chinese begin writing on silk

252 BCE         Dating of the oldest piece of paper ever found in Lu Lan, China

105 BCE         Cai Lun of the Chinese Han court is credited with inventing paper

256 CE            First known book on paper produced in China

500-600 CE   Mayans develop bark paper

610 CE            Korean monk takes papermaking to Japan

751 CE            Papermaking in Samarkand begins – they are credited for producing

high quality paper exclusively from (linen) rags

1264 CE          First record of papermaking in Fabriano, Italy – they are credited

with first using watermarks to identify the papermaker.

1309 CE          Paper is first used in England.

1495 CE          John Tate establishes the first paper mill in England in Hertfordshire.

1502-20 CE   Aztec tribute book lists forty-two papermaking centers.  Some villages

produce half a million sheets of paper annually.

1575 CE         Spanish build the first paper mill in Mexico.

1729 CE         Papermaking in Massachusetts begins.

1833 CE         An English patent is granted for making paper from wood.

1863 CE         American papermakers start using wood pulp.  (Kurlansky 337 – 3446)

History of Origami

The word origami comes from the ori- meaning, folded and –kami meaning, paper.  Although the Chinese developed papermaking, the Japanese developed the art of origami.  The first Japanese folds date from the 6^th Century A.D.  Since paper was scare and precious at that time, the use of origami was limited to ceremonial occasions.  The designs were limited to representations of animals, people, and ceremonial designs.  The designs were passed down from generation to generation, usually from mother to daughter.

Some of the oldest existing directions for paper folding were printed in Japan in 1797, entitled Sembazuru Orikata or Folding of 1000 Cranes.  You may be familiar with the story of the young Japanese girl, who contracted leukemia after World War II from the effects of the Hiroshima atomic blast.  The crane is a symbol of good luck in Japan and the tradition was that if you fold 1,000 cranes, you would be granted one wish.  Young Sadako Sasaki decided to fold 1,000 cranes so that her wish to get better would be granted.  She died before achieving her goal, 365 short.  After she died, her classmates folded the rest for her and placed them in her coffin.

Akira Yoshizawa is credited with making origami popular again.  He was born to dairy farmers on March 14, 1911 in Japan.  When he was 13, he had to take a job in a factory in Tokyo.  In his early 20s, he was promoted to “technical draftsman,” responsible for teaching new employees basic geometry.  He had learned origami as a child so decided to use it as a tool to help these employees understand geometry.

Yoshizawa quit his job in 1937 to practice origami full time.  He lived in poverty for close to twenty years and during World War II, he served in the army medical corps.  To cheer up the sick patients, he made origami models but eventually became sick himself and was sent home.  Finally in 1951, a Japanese magazine asked him to fold the twelve signs of the Japanese zodiac.  This exposure basically led to his fame.  In 1954, he founded the International Origami Centre in Tokyo and through his travels became a goodwill ambassador for Japan.  He died in 2005 at the age of 94.

In the 1960s, two origami societies were established:  The Friends of the Origami Center of America and the British Origami Society.  With the resurgence of interest in origami, it has evolved into different forms including modular folds, three-dimensional folds, folds that combine several subjects into a single fold, action figures, and figures that move when tugged.  I believe that one reason that origami has become so popular and so many new ways to fold paper have become popular is because of the variety of paper that is manufactured today.  I cannot imagine making action figures or modular folds with the coarse, thicker paper that was made years ago.  It is with new technologies and materials that our paper today can be as think or thick as we desire.  Origami paper can be purchased in square shapes with different colors or patterns on either side to make folding paper much easier and more precise than in times past.  Today, the concept of paper folding has also been used in health care, i.e. cardiac stents, and science, folding lenses to fit into spacecrafts that can be remoting unfolded once in space.  Science and technology has taken the concept of paper folding and used it to fold different materials such as plastic and metals to advance our ability to save a life or see farther into the universe.

Basics of Origami

Origami is the art of folding an uncut sheet of paper into an object and animal.  Yoshizawa  invented a systematic code of dots, dashes, and arrows that was adopted by western authors Harbin and Randlett in the early 1960s, which is still used today.

This standardized the techniques and terminology of folds that people around the world use – if you know the system, you can recreate the design even if the book is written in a different language.

The following general rules are given when creating an origami design:  students should work on a hard, smooth, and flat surface so that their folds can be accurate, it is important that each fold and crease be precise and that a pencil or thumbnail is used to move over the fold for exactness, study the diagram/instructions before folding the paper, and if students use colored paper, start with the colored side facing down at the beginning of their folding.

This system includes instructions using lines, arrows, and terms used to describe these series of lines and arrows.  There are five different types of lines: paper edges, either raw or folded, are drawn with a solid line. Creases are drawn as a thinner line and will often end before the edge of the paper.  Valley folds are drawn with a dashed line and mountain folds by a chain of dot-dot-dash line.  The X-ray line or dotted line when shown on a drawing indicates anything hidden behind other layers or represents a hidden edge, fold, or arrow. (Lang 2003, 15).

(Math in Motion, Pearl 41)

Geometry and Origami

Using origami to introduce more complex abstract concepts to elementary students is a way to allow students to comprehend shapes and angles.  We may think that all students understand that a square has four sides of the same length and four right angles, but I have seen the Ah-ha! Moments some fourth graders have when they make one from an 8 ½ by 11” sheet of paper.  Using folds or creases; students can create triangles, such as equilateral triangles.  Here again, do students really understand what an equilateral triangle is?  They will after completing an activity where they are asked to create one from a square.  Thomas Hull wrote in Project Origami: Activities for Exploring Mathematics that “…when choosing to use origami as a vehicle for more organized mathematics instruction, an easy choice is to let the students discover things for themselves.” (Hull xi).  Origami is a great strategy to use to help students discover properties of two-dimensional shapes.  Students can create hexagons, octagons, and nonagons by using origami.

Students in fourth grade have a difficult time understanding angles.  They see two rays coming together at a point.  They are shown a protractor and shown how to measure the angle created by the two rays.  Students spend time practicing these measurements with their protractors and terms such as right, acute, obtuse angles.  One way to help students to grasp these concepts and discuss where we use geometry in the world would be use a square sheet of paper is to point that one corner is a right angle and by making creases (folding) the paper, they can create different acute angles.

Students can also fold a square making two creases that intersect at the middle of the square.  A circle can be drawn around the intersecting point so that students can see when we divide a square into four sections, we are creating four right angles each representing 90 degrees or a right angle.  If we multiply 90 times 4, we get a product of 360 degrees, which represents the total degrees of a circle.

Geometry terms such as lines, points, angles, triangles, rectangles, etc. can be modeled and discussed as part of an origami lesson.  Asking students to identify these terms and create them will support students who are visual learners.  Another concept that can be modeled is patterning.  Students will be able to create patterns through paper folding, especially when creating three-dimensional shapes.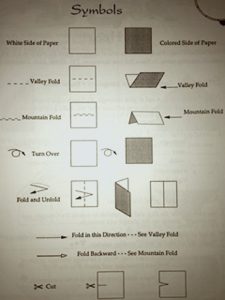

1. Students will be able to construct two-dimensional shapes in order to analyze the properties of two-dimensional shapes.
2. Students will be able to construct multi-step shapes in order to develop perseverance in solving problems.
3. Students will be able to construct three-dimensional shapes in order to analyze the properties of three-dimensional shapes.
4. Students will be able to analyze characteristics of two and three-dimensional shapes in order to develop mathematical arguments about geometric relationships.
5. Students will be able to develop basic geometric principles in order to construct and deconstruct models.

Developing authentic mathematical experiences for students is the best way to engage students and provide practice with abstract concepts.  Students will have an opportunity to answer questions and problems using origami.  For example, students will begin by folding two-dimensional four sided shapes into three to eight sided shapes.  They will discuss what they notice about constructing these shapes.  They will discuss what patterns they notice in the line creases they create when constructing each shape and what this tells them about the shape itself.

Teaching origami can seem daunting when you have a classroom of thirty plus students.  Folding paper into smaller and smaller shapes will be difficult for students to see.  Some will need one-on-one modeling while others will need to see the folds up close.  One strategy I will use to accommodate students is to use a document camera while identifying math concepts and terms and while constructing two and three-dimensional shapes.  Document cameras are a great way to model step-by-step instructions that will allow students to follow along.

Another strategy that I would like to try is to choose four to five students the meet with before beginning the unit.  I was thinking I would create an “origami club” where these students and I would meet once a week during lunch and construct origami shapes and become experts in creating the two and three dimensional shapes that we will be doing in class during the unit.  After presenting the lesson with the whole class, discussing concepts and modeling the origami shape, the experts along with the teacher will go around and assist the students who are struggling to construct the shape.

Another strategy that I would incorporate is for students to write reflections about their process.  After finishing an origami shape, students will be given prompts to reflect on in their “origami journal.”  Using mathematical terminology, students will be asked questions such as, what shapes did you notice when you unfolded your paper (creases in the unfolded paper will have different shapes).  What shapes do you notice in your finished three-dimensional shape?  What angles did you find when you unfolded your shape?  How many times did you have to unfold your paper and start again?  What part of making this shape was the most difficult?  Why?   Integrating writing with our lessons is important because students are asked to explain their answers in math.  Students can use the practice of writing about their thinking and learning in this unit as well.

Finally, to assess students’ understanding of the geometric terms and origami skills, I would use Exit Slips for each lesson.  Think of what you would like students to learn, for example, in the first lesson, using half sheets of paper, ask students to make two isosceles triangles and label the right angle.  For lesson two, ask students to define and draw horizontal and vertical lines.

These lesson plans are designed for fourth graders and can be modified to use for other grades.  By choosing other shapes and designs in the books under Teacher Resources, teachers in third, fifth, and even sixth grade can use these lesson plans.  I designed these lessons to be used for a period of five to ten days using days eight through ten as “enrichment” lessons where students can learn more complex designs found in the aforementioned books.  I found once I taught the first lesson on the history of origami and the basic terms, students couldn’t wait to create shapes and designs.

These lessons could be spread throughout a quarter as well.  You could designate Fridays for seven weeks, for instance, as “Origami and Math Day” to complete this unit.  The benefit of doing this is that students are given a week to practice their origami skills.  By introducing and reviewing geometric concepts each lesson, students gain a deeper understanding of abstract concepts.  You can extend the enrichment lessons for as long as you like throughout the year.  There are books in the resources that give many complex and fun designs to make.

I recommend that you choose three to four students who you know would love to have the role as “experts” for your lessons.  Meet with them for approximately fifteen minutes before each lesson, which is easier to do when teaching one lesson per week, and show them your next lesson.  Allow them time to practice by giving them paper to take home.  They will be great assets in assuring the success of your lessons as they walk around the room helping those who are struggling to complete the shape or design.

Finally, you will have to practice completing each one of these designs before teaching the lesson.  It will take some time to master the more complex shapes and designs.  Since each lesson includes modeling with a document camera, the more expert you are, the smoother the lesson will go.  Using origami paper while modeling is also helpful as the two sides, colored side and white, are easily seen on the Smart board.

Lesson One:  History of Origami and basic terms

Objective:  Students will be able to identify squares, rectangles, and isosceles triangles in order to create an origami shape.

Materials:

• document camera
• copy paper
• short history of Origami taken from the Background
• Origami Math – see resources
• Smart board

Vocabulary:

• fold
• crease
• mountain fold
• valley fold
• isosceles triangle

Procedures:

• Short History of Origami – origins given in the History of Origami above
• Why origami? Ask students to turn and talk to a partner about why origami would be beneficial to use in math lessons. Show images of origami used in science today.
• Basic terms – review and model symbols page given above: mountain, valley, crease, and fold, which is pictured under Basics of Origami. Or you can view this YouTube video on making a square: youtube.com/watch?v=gLrKrHgAI40.   Note to the students that the presenter is carefully folding and creasing his paper.
• Share Tips for success –patience, sharp and precise folds, perseverance, following step by step procedures
• Model and practice – have students make a square using a sheet of copy paper. Point out that the two triangles that are created when folding the top portion of the paper are isosceles right triangles. Define an isosceles triangle and start an anchor chart.  You can tape an example of an isosceles triangle to the chart.
• The strip of paper left over can be used to create a simple heart shape using rectangular strips of paper.  Use the procedure used on page 9 of Origami Math.  Or you can use this website to create a heart using origami paper.  youtube.com/watch?v=nnV262Egucw.  Students can design one side of the paper to make it their own. Students can also write a message to a friend, family member to write inside the heart and share it with them.
• Exit Slip-given a square sheet of paper, students will fold the paper to create two isosceles triangles and label the two right angles.

Lesson Two:  Geometric Shapes

Objective:  Students will be able to use a square sheet of paper to create a 3-dimensional pinwheel.

Materials:

• Square paper – 6” x 6”
• Instructions for a Basic Form, Net I, and Net II in the appendix
• Straw
• Pins or wire
• Document Camera
• Smart board

Vocabulary:

• Diagonal cross
• Grid lines
• Horizontal lines
• Vertical lines

Procedures:

• Review properties of a square, including four right angles and four equal sides. Although this may sound basic, asking students to turn and talk to discuss the difference between a rectangle and a square is a good way to check for understanding.  Introduce vocabulary words and add to the anchor chart in Lesson one.
• Model with students how to fold Basic I, a straight and diagonal cross using the document camera. This Basic form can be found on page 16 of Easy & Fun Paper Folding.  As students fold, refer to geometric shapes that the creases create.  Ask students what they notice about the shapes they are creating from the Basic I folds.
• Model with students how to fold Net I and Net II, which is a basic form for many shapes. These forms can be found on pages 17 and 18 of Easy & Fun Paper Folding.  Use the document camera again so students can see the folds and creases.  As students fold, ask them what they notice about the shapes the creases are creating.
• Use Basic form, Net I, and Net II to create a pinwheel in the appendix using the pinwheel design, straws, and pins/wires. Remind students to be careful with the pins.
• Exit Slip-given a square sheet of paper, students will fold to create horizontal and vertical lines and label each line.

Lesson Three:  Jumping Frog

Objective:  Students will be able to use origami procedures to create a moving 3-dimensional shape.

Materials:

• 3” x 5” index cards
• Math in Motion instructions on pages 52, 53, and 54.
• Document camera
• Smart board

Vocabulary:

• Line segment
• Intersect
• Perpendicular lines
• Pentagon
• apex
• Plane

Procedures:

• Introduce vocabulary words and add to the anchor chart from Lesson one. Students could draw these terms on the chart using a straight edge.
• Introduce motion/action origami – shapes that can be folded in a way that when “prodded” can move
• Use Pearl’s instructions on pages 53 and 54 using a document camera so students can watch each step. As you model the first time (students watch you make the entire frog and then you make it step-by-step together), use vocabulary term while modeling.  Or you can show this YouTube video which is about 10 minutes long:
• youtube.com/watch?v=PR_AI3CM2-A.
• Unfold your frog and model step-by-step instructions while students follow along. Allow students time to practice moving their frog.
• Ask students what other movable origami shapes they could make that would move. Allow students to work in pairs to design a shape that would move.

Exit Slip-students will draw perpendicular lines, intersecting lines, and line segments.

[Please see PDF above for additional activities & appendices]

The following represent Pennsylvania standards for fourth graders:

CC.2.3.4.A.2  Classify two-dimensional figures by properties of their lines and angles.

CC.2.3.4.A.3  Recognize symmetric shapes and draw lines of symmetry.

CC.1.5.4.A  Engage effectively in a range of collaborative discussions on grade-level topics and texts, building on others’ ideas and expressing their own clearly.

Boakes, Norma. “The Impact of Origami-Mathematics Lessons on Achievement and Spatial Ability of Middle-School Students.” Origami 4, May 2009, pp. 471–481., doi:10.1201/b10653-46.  

This article discusses ways to use origami in the mathematics classroom.  Ideas are given to educators and results of studies completed on the spatial ability of middle school students.

Hull, T. (2006). Project Origami: Ideas for Exploring Mathematics. Wellesley, PA: A.K. Peters.

This is a technical book for teachers who are looking for more complex origami designs along with ideas for math lessons for older students.  There are many suggestions for lessons plans and essential questions for students to write their reflections.

Kurlansky, M. (2016). Paper: Paging through history. New York: W.W. Norton & Company.

This book presents the history of paper tracing the origin of paper from papyrus, silk, and animal skins to paper making in early America.  The author presents background information on how the idea of writing on different types of paper evolved throughout time.

Lang, R. J., & Macey, R. (1988). The complete book of origami: Step-by-step instructions in over 1000 diagrams: 37 original models. New York: Dover.

For teachers who would like more complex designs to make with their students, this book offers 37 complex designs with step-by-step instructions including elephants, scorpions, tarantulas, etc.

Lang, R. J. (2003). Origami Design Secrets: Mathematical Methods for an Ancient Art. Natick, MA: A.K. Peters.

This book is a comprehensive guide to making complex origami designs.  It includes basic instructions, crease patterns, and step-by-step instructions for many animals.